| Pros | Cons |
|---|---|
| You don't give away ownership | If you can't afford it, you can't do it |
| You don't need to pay debt obligations | |
| You don't have to pay back the money | You give away some ownership |
| You share the risk with your investors | Need to find investors |
| Less stress to make immediate profit | Developing shares can be complicated and expensive |
| Your investments are more profitable (leveraging) | |
| You keep ownership | You pay interest on your loan |
| Interest on loans is tax-deductible | You have additional risk if you are slow in paying it back |
| Your investments are more profitable (leveraging) | You have to have credit established previously |
4 Financial Project Evaluation
For which of you, intending to build a tower, sitteth not down first, and counteth the cost, whether he have sufficient to finish it? Lest haply, after he hath laid the foundation, and is not able to finish it, all that behold it begin to mock him, Saying, This man began to build, and was not able to finish. Luke 14
Infrastructure takes money to build. Money is needed to pay for the people to plan and build projects including tradesmen, construction managers, and engineers. Money is also needed for things such as building supplies, construction equipment and land on which the project is to be built. How can you know if the money you spend on a project will be worth it? How can you decide which version of a project to build? How can you deal with the fact that money now is worth more than money later?
Private businesses will evaluate a construction project as to whether it can return a reasonable profit. Governments may choose to build a project that provides the most benefits for their citizens at the lowest cost. Either way, a well-informed business, non-profit organization, or government will conduct a financial analysis of a potential construction project before committing to it. In addition, a financial analysis can guide planners, engineers, and construction managers on the appropriate design, scope, timeline, and resulting costs of an infrastructure project.
Think
Many of the principles and techniques you learn in this unit can apply to your own life, despite the meaningful differences between running a government or business and running a home. President Gordon B. Hinckley cautioned,
I recognize that it may be necessary to borrow to get a home, of course. But let us buy a home that we can afford and thus ease the payments which will constantly hang over our heads without mercy or respite for as long as 30 years. … I urge you, brethren, to look to the condition of your finances. I urge you to be modest in your expenditures; discipline yourselves in your purchases to avoid debt to the extent possible. Pay off debt as quickly as you can, and free yourselves from bondage.
Important
Many of the terms we use in this unit are used casually or differently by individuals, businesses, and industries (Boyte-White 2024). In this class, we will try to be consistent with our use of these terms. As a professional, it will be your responsibility to understand how each term is defined when evaluating financial analysis of a project or when communicating your results to others.
If you are unfamiliar with a term, look it up! Or ask! There are many good credible resources online. For example, see the Investopedia Dictionary (Investopedia 2024) and the U.S. Consumer Financial Protection Bureau glossary (US CFPB 2024a).
As always, you should know definitions for the terms in bold.
4.1 Returns and Investments
Whenever you have a financial project — this can be something as basic as a savings account or as complex and large as a multi-year heavy civil construction project — you are hopeful that what you get out of the project will be worth at least as much as you put into it. After all, if you don’t get more money than you started with, why even do it? You might as well have put your money in a piggy bank.
What we put into a project is called its investment. What we get out of a project are called its returns. Mathematically, the returns are
\[ \mathrm{Returns} = \mathrm{Revenue} - \mathrm{Expenses} - \mathrm{Interest} - \mathrm{Taxes} + \Delta(\mathrm{Asset\ Value}) \tag{4.1}\]
Each of these terms in Equation 4.1 deserves a definition.
- Revenue is the money earned from a project.
- Expenses are money spent during a project in order to operate it.
- Interest are payments that are required by any debt associated with the project.
- Taxes are payments to the government required as part of a project’s operations or profits.
- Assets are resources associated with the project. The value of an asset might go up or down based on market conditions, improvements that are made to the asset, or the loss of the asset’s usefulness.
Any positive return is better than a loss, but a small positive return on a massive investment would be disappointing. A measure the effectiveness of an investment is return on investment or ROI. This is simply the ratio of returns to investments, or
\[ ROI=\frac{\mathrm{Returns}}{\mathrm{Cost\ of\ Investment}} \tag{4.2}\]
We often describe ROI as a percentage.
Payback Period
Another basic measure of the effectiveness of an investment is the payback period. This is the inverse of ROI if the returns are in per-year increments.
\[ \mathrm{Payback}[\mathrm{time}] = \frac{\mathrm{Investment \$}}{\mathrm{Returns} \$/\mathrm{time}} \tag{4.3}\]
Let’s explore the other parts of the Returns equation in Equation 4.1. If you have operating expenses or are collecting revenue from your project, you need to account for that in your calculations of the returns.
Expenses vs Investments
What is an expense and what is an investment? Expenses and investments in a project each reduce the returns in Equation 4.1, so why does it matter where you put them? When you are calculating the taxable income on your project, you can often deduct expenses, but you cannot usually deduct investments.
Where you put investments and where you put expenses also affects the ROI. In the prior example, the sewer line repair is an expense of operating the project. If you had preemptively fixed the sewer line, then it would have counted as part of your initial investment; you would have had the same returns but a lower ROI because your investment was bigger.
So pay attention to where you are putting what!
What happens when you use debt to start a project? This will decrease the returns from the project, because you will have to pay interest to the person who lent you the money. The amount you pay in interest in a period is usually the interest rate given with the loan multiplied by how much you borrow:
\[ \mathrm{Interest} = \mathrm{Interest\ rate}\times{\mathrm{Principal}} \tag{4.4}\]
Warning
Interest rates are usually given in percentages (12%), but should be used as decimal proportions in equations (0.12).
But you won’t have to invest as much in the project to start with, because that money isn’t yours. This can substantially increase the profitability of the project.
This process of using debt to increase the profitability of a project is called leveraging. There are of course pros and cons associated using debt in this way. The returns go down, meaning that doing one project with debt would be a little less profitable than doing one project without it. But because you have less of your own resources invested in that one project, you could maybe do two projects! In that way you can increase your total overall returns across for the same amount of investment. That’s why ROI is a good measure.
The cons of using debt to finance a project come when the project is unsuccessful. What if you don’t find renters? Or the sewer repair costs $50000? In that case, you don’t just lose your investment; the bank loses theirs also and you might not be able to borrow again on advantageous terms.
There are three general ways to finance a project, two of which we have already introduced. Table 4.1 gives a simplified summary of the pros and cons of each method, adapted from Hayes (2023). But to summarize,
- Self-finance Self-finance means to pay with your own money, or your business’s money.
- Debt-financing Borrowing money from a bank, person or another institution. Lenders need to be paid back the borrowed amount plus interest.
- Equity-financing Selling ownership of your business to fund business operations. Equity is another word for ownership in a company.
4.2 Taxes
Taxes are “required payments of money to governments, which use the funds to provide public goods and services for the benefit of the community as a whole.” (US CFPB 2024b). In the private sector, you need to account for tax payments when you consider the profitability of your projects. In the public sector, taxes provide funding and incentivize the behavior of private sector actors.
As a construction manager or engineer, you should rely on certified accountants to understand the details of the tax code, provide advice you on financial decisions, and/or file your taxes. However, a basic understanding of taxes will help you make wise business and infrastructure investment decisions. In this section, we will introduce basic tax principles related to businesses and infrastructure projects.
The tax a company pays is the product of the tax rate and the taxable income. \[ \mathrm{Taxes}=\mathrm{Taxable\ Income} \times \mathrm{Tax\ Rate} \tag{4.5}\]
Effective tax rates
In the US, most companies are required to pay both state and federal taxes. Federal tax code generally allows state taxes to be deducted from federal taxes, however. The effective tax rate a company pays combines both of these rates into a single number,
\[ \mathrm{Effective\ Tax\ Rate}=\mathrm{State\ rate}+(1-\mathrm{State\ rate})\times(\mathrm{Federal\ rate}) \tag{4.6}\]
In this class, you should assume that tax rates given in problems are effective tax rates unless told explicitly otherwise. Common corporate effective tax rates are on the order of 25% to 40%, depending on industry and state.
Note that Equation 4.5 relies on a concept of taxable income, which is defined as
\[ \mathrm{Taxable Income} = \mathrm{Revenue} - \mathrm{Expenses} - \mathrm{Interest} - \mathrm{Depreciation} + \mathrm{Capital\ Gains} \tag{4.7}\]
Note how similar Equation 4.7 is to Equation 4.1. In fact, taxable income is meant to be based on the returns of your project. The one exception is related to the change of value in capital assets — objects that hold value like property, equipment, or company stock.
- If your asset loses value, you can deduct this loss from your taxable income. This called depreciation.
- If an asset you owned for more than one year gained value, you have to pay taxes on these capital gains in the year you sold it.
Capital gains tax rates
Capital gains are usually taxed at a lower rate than income, for both corporations and for individuals. This incentivizes people to hold onto assets rather than selling them quickly, which can be bad for an economy. On the other hand, it can lead to distortionary behavior. Many company executives take their compensation in stock, and then pay a lower tax rate than if they were simply paid a salary.
In this class, we will just use a single effective tax rate.
4.2.1 Depreciation
When you have a capital asset that depreciates in value, you can deduct the loss of that value from your taxable income as shown in Equation 4.7. This encourages companies to invest in new equipment and facilities, because they can recover their costs over time. There are two general methods to calculate the depreciation in a given year:
- Straight-line depreciation assumes that the asset loses equal value in each year.
- Declining balance depreciation assumes that the asset loses an equal proportion of its remaining value in each year.
The sraight-line depreciation method assumes that the depreciation \(D\) in each year \(t\) is: \[ D_t=\frac{C-S_n}{n} \tag{4.8}\] Where
- \(D_t\): depreciation in year \(t\)
- \(C\): initial cost
- \(n\): expected life of asset
- \(S_n\): expected salvage value in year \(n\)
The declining balance method relies on factors — shown in Table 4.2 — to calculate the depreciation \(D\) in a given year \(t\):
\[ D_t=C\times d_{t} \tag{4.9}\]
Where
- \(C\): initial cost
- \(d_{t}\): depreciation rate for year {t}
Note that an asset with a ten-year depreciation timeline has eleven factors. This is because the factors assume you buy the asset in the middle of the year you buy it, and finish depreciating it halfway through the last year. Details on how the factors are calculated are in the expandable callout below.
| Year (t) | 3 | 5 | 7 | 10 |
|---|---|---|---|---|
| 1 | 33.33 | 20 | 14.29 | 10.00 |
| 2 | 44.45 | 32 | 24.49 | 18.00 |
| 3 | 14.81 | 19.2 | 17.49 | 14.40 |
| 4 | 7.41 | 11.52 | 12.49 | 11.52 |
| 5 | 11.52 | 8.93 | 9.22 | |
| 6 | 5.76 | 8.92 | 7.37 | |
| 7 | 8.93 | 6.55 | ||
| 8 | 4.46 | 6.55 | ||
| 9 | 6.56 | |||
| 10 | 6.55 | |||
| 11 | 3.28 | |||
| Factors given as percent values. |
Declining Balance
Why is it called the declining balance method?
Sometimes businesses might be reluctant to invest in capital projects — buildings, warehouses, heavy equipment — because capital investment is not excluded from taxable income. This would slow down the economy as people work in less efficient spaces and with older machinery.
To encourage more capital investments by private industry, the US introduced the use of accelerated depreciation methods as part of the Modified Accelerated Cost Recovery System (MACRS). This allows businesses to recoup more of their capital assets through depreciation faster than the straight line method would allow.
The accelerated depreciation methods used in MACRS uses a form of the declining balance (DB) method. In the declining balance method, the depreciation is calculated as a fixed percentage of the remaining book value. For example, in the 200% declining balance method, percent depreciation is calculated as \(d = \frac{200\%}{recovery\ period}\). For a 10-year recover period, \(d =\frac{200\%}{10}= 20\%\). The depreciation is calculating as a percentage of the book value at the end of the previous year: \(D_t = d\times{BV_{t-1}}\)(Blank and Tarquin 2023a).
Figure 4.1 compares the normalized book value, \(BV_t/C\), for the straight-line method, a true 200% declining balance method and the MACRS 200% declining balance method over a 10-year return period. Using a true declining balance method the book value will never reach zero. Thus, the MACRS 200% declining balance method switches to a straight-line method when the straight-line results in a larger depreciation than the declining balance method. In a 10-year recovery with a 200% declining balance, this occurs at age 6 as shown in Figure 4.1.
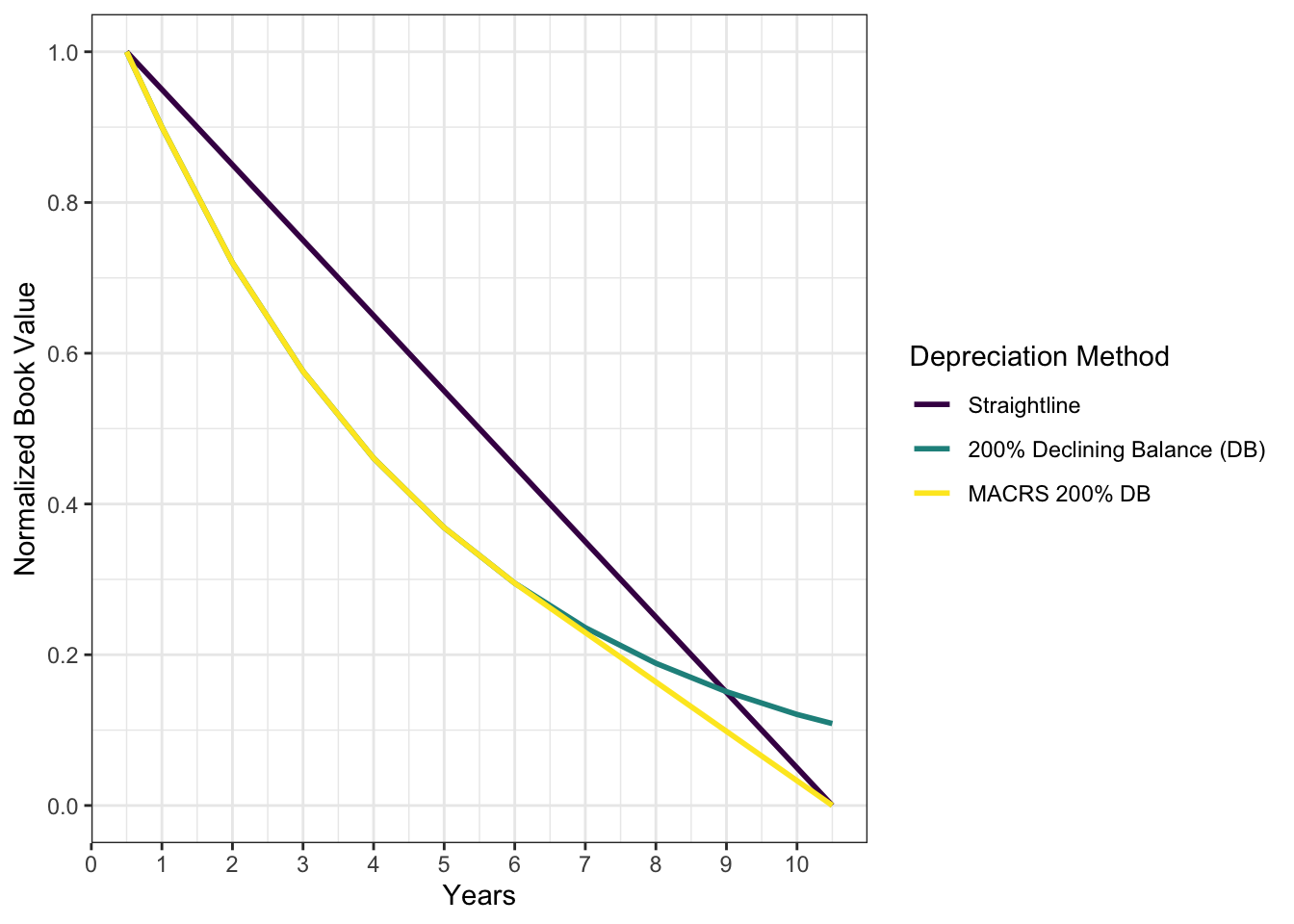
The IRS (2024b) simplifies the depreciation calculations by providing tables of MACRS depreciation rates, \(d_{t,p}\), by different methods, years \(t\), recovery periods \(p\), and for the season when the asset began service. In addition, MACRS requires the book value to depreciate decrease all the way to $0 at the end of the recovery period for both straight-line and declining balance methods.
Note there are 11 years of depreciation factors for a 10-year recovery period in Table 4.2. This is because a half-year convention is used, meaning that we assume the asset is placed into service half-way through the first year \((t=0.5\ years)\), and ends half-way through the eleventh year \((t=10.5\ years)\) as shown in Figure 4.1. The first and eleventh year depreciation factors are 50% smaller than if they were full years.
In this course we provide depreciation factors for the MACRS 200% declining balance method using the half-year convention in Table 4.2. All the homework and exam problems regarding MACRS will use this table. The IRS (2024b) dictates the recovery periods for different classes of assets.1 The recovery period will be given you in your homework and exam problems. In your career you will need to consult IRS (2024b) or a certified accountant.
MACRS
Technically, MACRS defines both the straight-line and various declining balance methods depending on rates of depreciation and the seasons when the asset enters service, but many sources — including FE and PE reference manuals — use MACRS to refer to one particular declining balance method only. As in, they define two methods, straight-line recovery and MACRS. Pay attention to the context of the problem!
An important concept related to depreciation is the book value of the asset, or how much an asset contribute’s to your company’s overall value in a particular year \(t\). This is defined as
\[ BV_t=C-\sum_{n=1}^t{D_n} \tag{4.10}\]
Where
- \(BV_t\): Book Value in year \(t\)
- \(C\): initial cost
- \(n\): number of years in recovery period
- \(D_n\): depreciation in year \(n\)
Note that the book value is different from the market value — what the asset would likely sell for. For example, a the market value of a warehouse could appreciate from the purchase price due to rising real-estate prices, but for tax purposes the book value could decrease from the initial value, allowing the owner to take tax deductions on the decline in value of the warehouse. (Blank and Tarquin 2023a).
Which depreciation method should I use for my project?
The declining balance method will allow you claim larger deductions from your investments sooner. You can then use the money you save in taxes to recoup the costs of your investment, help pay off your loans of your capital investment, or invest in other projects.
If you have many other tax deductions in the earlier years of the recovery period, you may elect for one of the other methods that gives you larger tax deductions in later years of the recovery period.
4.3 Time Value of Money
Infrastructure is expensive to build, but it generates value and benefits over a long period of time. Because populations and economies grow, the opportunity cost of not building infrastructure is high. As a result, virtually all infrastructure is built using financial tools that harness something called the time value of money. These tools allow companies or governments to concentrate many years of revenues into a short period to build or purchase something expensive; at the same time, these tools allow investors to grow their monetary reserves and profit from the responsible development of society.
For the examples given in the first section, we generally limited our analysis years to one year returns. When considering projects over larger time periods, it is important to consider the time value of money.
Imagine you win a contest and are given two options. You can choose to collect your $1,000 prize today, or you can wait for ten years to get the same amount of money. Which option would you choose? There are at least three reasons you should take the money right away:
- Risk There is a possibility that something bad could happen to your prize. Within the next 10 years, the company offering the prize could go bankrupt. Or you could die or become otherwise disqualified from claiming the prize.
- Inflation Money loses value over time for a variety of reasons. $1000 will buy more goods and services now than it will in ten years.
- Opportunity cost If you got the prize now, there are things you could do with it. You could spend the money on something that you would enjoy for the next ten years. You could also invest the money for ten years, during which time you would earn on that investment. Getting the prize later costs you these opportunities.
Money has value. Having money now is more valuable than having money in the future. This is called the time value of money. If I were to lend you money, I would want to receive not only the initial loan amount, but some payment to compensate me for the risk, inflation, and opportunity cost of making the loan. This payment is called interest, as we saw in Equation 4.4. When financial analyses extend for more than period (years), calculating interest becomes slightly more challenging. There are two basic methods:
- Simple interest loans require a defined interest payment on the principal of the loan, or how much the loan is for.
- Compound interest loans combine the accrued interest with the unpaid principal at a particular compounding rate.
The amount we will pay in the future \(F\) using a simple interest loan over multiple periods is: \[ F_\mathrm{simple}= P + P\times{i}\times{n} \tag{4.11}\] where \(P\) is the principal, \(i\) is the interest rate (usually percent per year), and \(n\) is the number of interest periods (usually years).
While simple interest loans are easy to calculate, almost all investments and loans use compound interest.
The amount we will pay in the future \(F\) on a compound interest loan is a requires a little derivation. After one period, the compound interest formula looks just like the simple interest one, \[ F = P + P\times i=P(1+i) \tag{4.12}\] If we have two periods, then we need to take the value from Equation 4.12 and multiply it by \(i\), plus add another Equation 4.12 again for the new interest we add in year 1 \[ F = P(1+i)+P(1+i)i=P(1+i)(1+i)=P(1+i)^2 \tag{4.13}\] If we keep doing this for \(n\) periods, we can derive a general equation \[ F = P(1+i)^n \tag{4.14}\]
4.3.1 Compounding Frequency
One consideration with compound loans is the compounding frequency, or how often the interest is applied into the total amount owed. Usually, the compounting frequency and the interest rate and the number of periods will all be the same: that is, \(n\) will be in years and \(i\) will be in percent per year, with annual compounding.
But that might not always be the case! Sometimes loans could compound more frequently than once per year. Many mortgages, for example, give a nominal interest rate per year, but actually compound monthly. In this case, Equation 4.14 becomes
\[ F=P[1+(i/k)]^{nk} \tag{4.15}\]
with \(k\) the number of compounding periods in each \(n\). You can see how this works: the nominal interest rate is divided across \(k\) periods, but the total number of periods multiplied to \(nk\). \(i/k\) is referred to as the periodic interest rate.
Compounding frequency
The compounding frequency has a pretty important role in how much the loan is worth in the future. Let’s look at three situations where $1000 is loaned at 10% interest (\(i = 0.1\)) for ten years.
- Yearly compounding (\(k\)=1): \(\$1000[1+(0.1/1)]^{(1*10)}=\$2593.74\)
- Monthly compounding (\(k\)=12): \(\$1000[1+(0.1/12)]^{(12*10)}=\$2707.04\)
- Daily compounding (\(k\)=365): \(\$1000[1+(0.1/365)]^{(365*10)}=\$2717.91\)
Figure 4.2 shows the growth in the loan amount at each compounding period for different compounding frequencies. There is obviously a big difference between annual and monthly compounding, but there isn’t that much difference between monthly and daily, or daily and even hourly compounding. This suggests that there is a limit to this function as \(k\) increases.
Continuous compounding
It turns out that if you take the limit \(\lim_{k\to\infty} F=P[1+(i/k)]^{nk}\) you can derive the equation for continuously compounding interest, \[ F=Pe^{in} \tag{4.16}\]
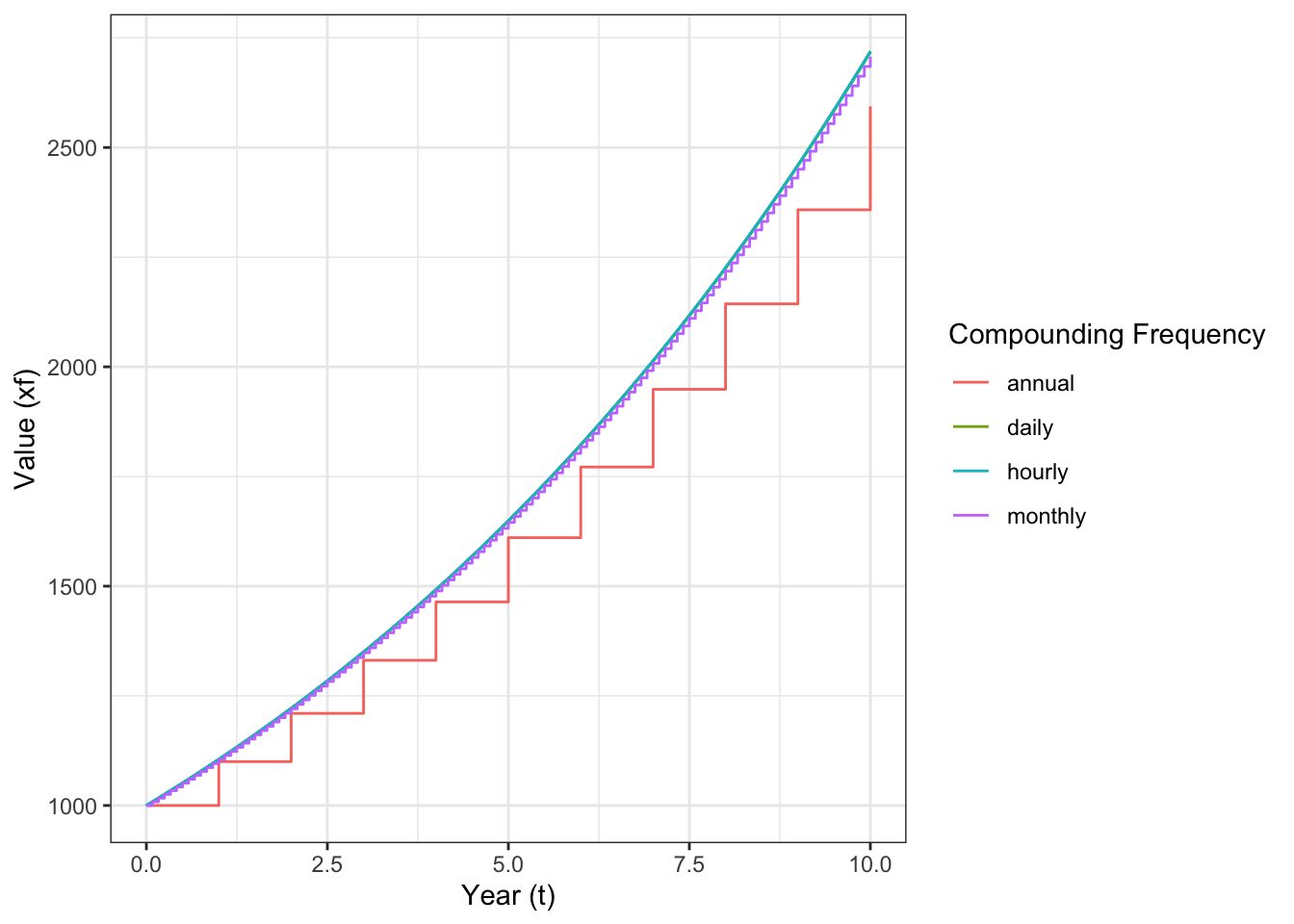
Using our new equation, we can find out how much money we will have after 10 years of continuously compounding the interest from our $1,000 invested at an interest rate of 10%.
- Continuously: \(\$1000*e^{(0.1*10)}=\$2718.28\)
Notice that this value isn’t significantly more than the amount of interest earned by compounding the interest daily. As the number of compounding periods continues to increase, the interest earned in each period increases by a diminishing amount. However, remember that even when the difference may be small, the more frequent the compounding period, the more interest is generated.
You may see bank advertising their savings accounts with an Annual Percentage Yield (APY) instead of an interest rate (Chen 2024). The APY is an annual interest rate compounded once per year that would give you the same returns as the actual or period interest rate using the compound frequency of the bank.
So after one year (\(n = 1\)), the future value of the investment using the APY and the actual interest rate and compounding frequency should be equal to each other:
\[\begin{align*} F_{\mathrm{annual}} &= F_{\mathrm{actual}} \\ P(1+APY)^n &= P(1+i/k)^{nk} \\ P(1+APY)^1 &= P(1+i/k)^{k*1} \end{align*}\] \[ APY = (1+i/k)^k -1 \tag{4.17}\]
The APY allows investors to compare returns from investments that have different compound periods.
4.4 Annuities and Gradients
Besides future and present values, two additional values might play a role in financial project planning and consideration. The first of these values is the annuity, \(A\). This is the equal periodic payment that will repay a loan over time, or the equal period revenue expected from an investment. If we pay it all off in one period, then \(F = A\). If it takes two periods, then the equation is \(F = A + A*(1 + i)\) including interest paid in the second period. Extending the full series \(n\) times and solving for \(A\),
\[\begin{align} F &= A + A*(1 + i) + A* (1 + i)^2 + \ldots + A*(i+1)^{n-1} = \sum_{j = 0}^{n -1} A ( 1 + i)^j\\ F(1 + i) &= \sum_{j = 1}^n A ( 1 + i)^j \\ F(1 + i) - F &= \sum_{j = 1}^{n } A ( 1 + i)^j - \sum_{j = 0}^{n-1} A ( 1 + i)^j \\ F+ Fi - F &= A*(1 + i)^n - A\\ Fi &= A*[(1 + i)^n - 1]\\ \end{align}\]
This finally reduces to \[ F = A*\frac{(1 + i)^n - 1}{i} \tag{4.18}\]
To turn a future value into an equivalent annuity, we just take the inverse.
\[ A=F*\frac{i}{(1+i)^{n}-1} \tag{4.19}\]
We could also derive equivalence between the annuity and its present value.
\[ P=A*\frac{(1 + i)^n -1}{i (1 + i)^n} \tag{4.20}\]
\[ A=P*\frac{i ( 1 + i) ^n}{(1 + i)^n - 1} \tag{4.21}\]
4.4.1 Gradients
The second value is a gradient, or an annuity that increases its value over time by a uniform amount \(G\)2. That is if \(G = 100\), then \(G_1 = 0, G_2 = 100, G_3 = 200, \ldots, G_n = (n-1)*G\). This can happen if, for instance, you expect that a business in operation will grow in each period, or if you plan to pay a larger amount on your mortgage as your income increases. We won’t do the derivation of this value, but the equations are:
\[ P=G*\left[\frac{(1+i)^{n}-1}{i^2(1+i)^{n}}-\frac{n}{i(1 + i)^n}\right] \tag{4.22}\]
\[ F=G*\left[\frac{(1+i)^{n}-1}{i^2}-\frac{n}{i}\right] \tag{4.23}\]
\[ A=G*\left[\frac{1}{i}-\frac{n}{(1+i)^n-1}\right] \tag{4.24}\]
Example
Maintenance on an old machine is $100 this year but is expected to increase by $25 each year thereafter. What is the present worth of five years of maintenance? Assume an interest rate of 10%.
This is an interesting problem because it involves both an annuity ($100 per year) and a gradient of $25 each year. The cash flow diagram looks like this:
We can then calculate the present value of each element separately and add them together,
\[\begin{align*} P&=G*\left[\frac{(1+i)^{n}-1}{i^2(1+i)^{n}}-\frac{n}{i(1+i)^{n}}\right]\\ &=25*\left[\frac{(1+0.1)^{5}-1}{(0.1)^2(1+0.1)^{5}}-\frac{5}{0.1(1+0.1)^{5}}\right]\\ &=172\\ P&=A*\frac{(1+i)^{n}-1}{i(1+i)^{n}}\\ &=100*\frac{(1+0.1)^{5}-1}{0.1(1+0.1)^{5}}\\ &=379 \end{align*}\]
for a total present value of $551
In all of our equivalence equations to this point, we necessarily assume some end-point \(n\) time periods in the future. But what about the equivalent present value of a perpetual benefit? We may use this assumption when considering the worth of capital investments where we don’t know when the benefits will end; if we want to find the present worth of this endless series of payments, we can use a simple formula for capital worth
\[ P=\frac{A}{i} \tag{4.25}\]
4.4.2 Calculation Shortcuts
It is important to know that the equations we have discussed so far exist, and that they are not magical constructs banks designed to part you from your money. But the equations themselves are somewhat unwieldy and impractical, and they are certainly difficult to remember. There are two primary shortcuts that you should be aware of.
- Factor tables
- Preexisting or custom software functions
4.4.2.1 Factor Tables
On the Fundamentals of Engineering (FE) exam and the exams in this class, you will not have access to Excel or to a programmable calculator. It is impractical to type in every element of each formula repeatedly; you are bound to make several mistakes.
Notice though that each equation we have looked at is the product of a scalar (\(A\), \(F\), etc.) and some factor of the interest rate and the time period. Watch what happens when we take a set of present values increasing by factors of 10 at a constant interest rate and time period.
for(P in c(1, 10, 100)){
# F = P * (1 + 0.1)^10
print(ptof(P, i = 0.1, n = 10))
}[1] 2.593742
[1] 25.93742
[1] 259.3742Unsurprisingly, the future values are direct multiples of the present value. This means we could create a table that contains the factors for different interest rates and periods. A partial table for 2% interest is in Table 4.5, and a complete set of tables are in Appendix A. The way to read these tables is as follows:
- Identify the table for the interest rate you are given.
- Determine which column of factors you need. You read \(F/P\) as “\(F\) given \(P\)”, so this is your column if you have been given a present value and wish to find the future value.3
- Find the row in the table for the given number of periods. If \(F/P\) is your column and the number of time increments is 5, your value is 1.1041.
- Multiply the original given value by the value from the table. Keeping with the same example, if you were given 1,000 dollars for \(P\), you multiply it by 1.1041 to find \(F\) = 1,104.10 dollars.
| n | P/F | P/A | P/G | F/P | F/A | A/P | A/F | A/G |
|---|---|---|---|---|---|---|---|---|
| 1 | 0.9804 | 0.9804 | 0.0000 | 1.0200 | 1.0000 | 1.0200 | 1.0000 | 0.0000 |
| 2 | 0.9612 | 1.9416 | 0.9612 | 1.0404 | 2.0200 | 0.5150 | 0.4950 | 0.4950 |
| 5 | 0.9057 | 4.7135 | 9.2403 | 1.1041 | 5.2040 | 0.2122 | 0.1922 | 1.9604 |
| 8 | 0.8535 | 7.3255 | 24.8779 | 1.1717 | 8.5830 | 0.1365 | 0.1165 | 3.3961 |
| 10 | 0.8203 | 8.9826 | 38.9551 | 1.2190 | 10.9497 | 0.1113 | 0.0913 | 4.3367 |
| 25 | 0.6095 | 19.5235 | 214.2592 | 1.6406 | 32.0303 | 0.0512 | 0.0312 | 10.9745 |
| 50 | 0.3715 | 31.4236 | 642.3606 | 2.6916 | 84.5794 | 0.0318 | 0.0118 | 20.4420 |
4.4.2.2 Functions
In practice, you will probably use functions that pre-exist in Excel or that you code yourself into software that you write. The examples on this page use functions that I wrote in the R programming language. For example, we can write a function for Equation 4.15 that converts \(P\) to \(F\), and then use that function to quickly find the future value for each of the compounding rates in the the example above:
#' Function to find F given P
#' @param P Present value
#' @param i Interest rate
#' @param n Number of periods
#' @param k Compounding iterations per period, default is 1
ptof <- function(P, i, n, k = 1){
P * (1 + (i / k) )^(n * k)
}
for(k in c(1, 12, 365, 365 * 24)) {
# print a message with how many times per year
print(
str_c("Compunding ", k, " times per year: ",
# 1000 for ten years at 10%, compounding k times per year
ptof(1000, 0.1, 10, k), sep = "")
)
}[1] "Compunding 1 times per year: 2593.7424601"
[1] "Compunding 12 times per year: 2707.04149086224"
[1] "Compunding 365 times per year: 2717.90955457697"
[1] "Compunding 8760 times per year: 2718.26631329575"In Excel, there are an array of functions that you can use. For example, the FV() function will compute the future value of an investment given either an initial value or a regular payment. That means, the FV function works both as Equation 4.15 and also as Equation 4.18. The results and use of this function are shown in Figure 4.3.
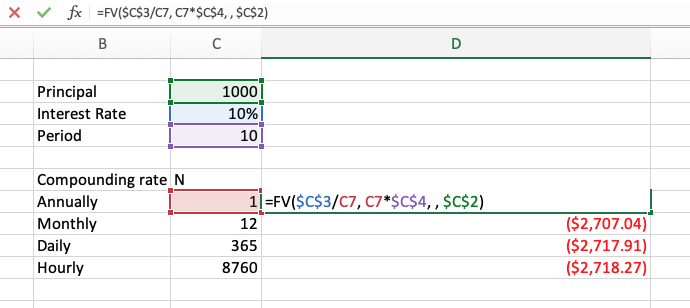
Table 4.6 Provides a lookup table of factors and their Excel and Google Sheets function equivalents.
| Factor | Excel / Sheets Function |
|---|---|
| P/F | PV(i, n, ,F) |
| P/A | PV(i, n, A) |
| P/G | Not included |
| F/P | FV(i, n, , P) |
| F/A | FV(i, n, A) |
| A/P | PMT(i, n, P) |
| A/F | PMT(i, n, , F) |
| A/G | Not included |
4.4.3 Financial Tools
Now that we have a basic understanding of the time value of money and the power of interest, we can discuss some of the tools that are often used to finance projects. These financial tools are based on the time value of money, and work by using the power of interest to raise the funding necessary for projects.
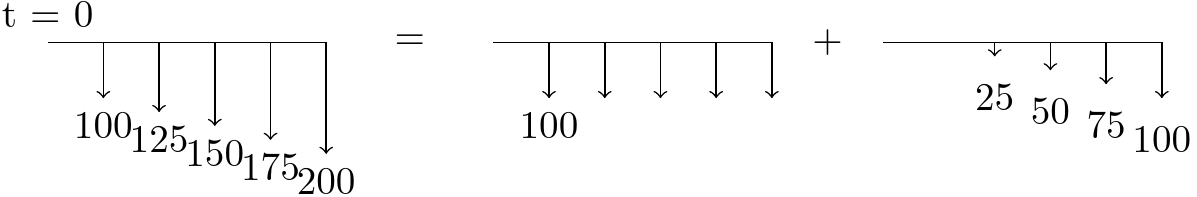
Financial tools differ based on how the loan is structured (interest, compounding), whether the project can bring in revenue while it operates, and when and how the loan is paid off. Each of these variables can be difficult to describe. A cash flow diagram is a chart that shows inflows and outflows of cash over time for a project or company, and can be a way to simplify these complex exchanges.
First, imagine that you have taken out a student loan of $10,000 at a 5% interest rate. You want to pay this back over the next 10 years, so you decide to pay it off by putting $1250 towards the loan every year until the final year, when you will pay it off in full. This cash flow can be represented with the diagram in Figure 4.4.
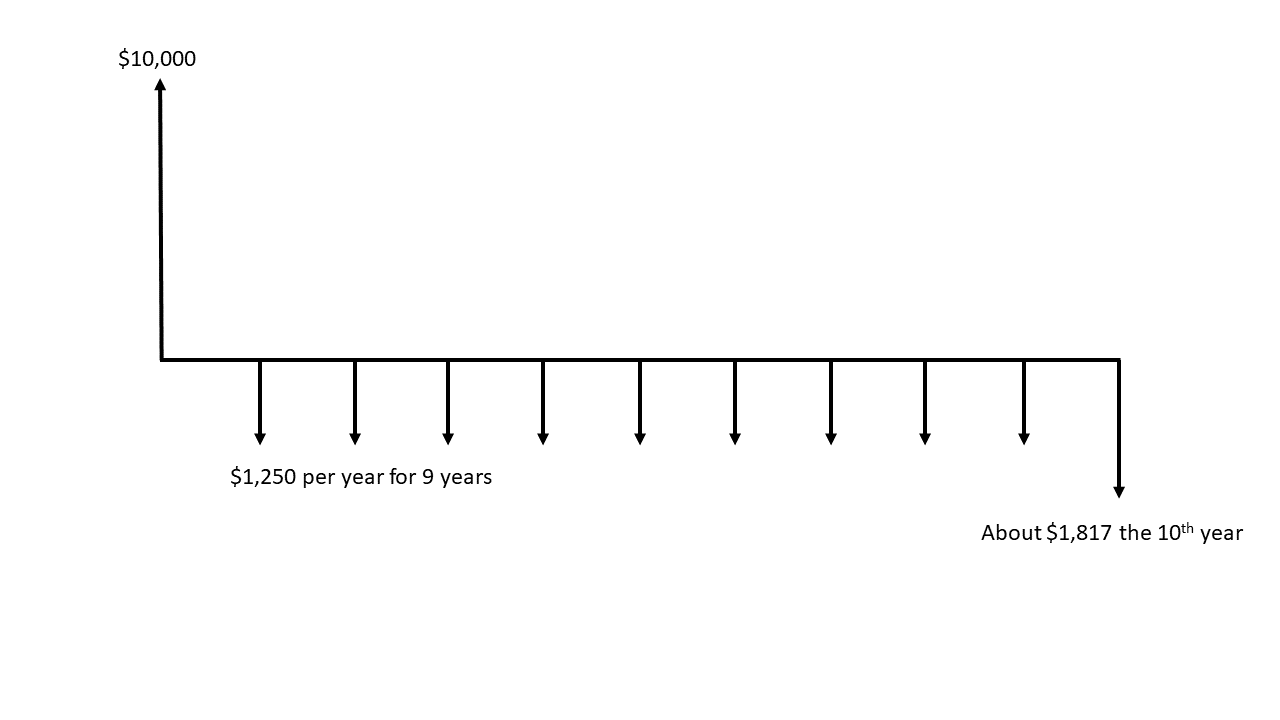
The diagram is set up by drawing a horizontal line representing the time over which the cash flow takes place. Intervals represent periods of time, in this case years, starting at 0 when you initially borrow the $10,000. Arrows that point up show cash inflows, like the $10,000 you borrowed initially. Arrows that point down show cash outflows, like the payments you make over the next 10 years to pay back the loan. Notice that larger amounts have larger arrows, although they don’t need to be exactly proportional. Another important point is that each arrow represents the net cash flow for each period of time. In some cash flow diagrams you may have inflows and outflows during the same period, but they can be added together and represented using just one arrow.
Note that the borrower and the lender will each have their own cash flow diagram, and that these diagrams will be symmetrical. Let’s look at a couple of examples of cash flow diagrams. Let’s look at the diagram for the bank that loaned you the $10,000, shown in Figure 4.5. Because they lent the money, their cash flow diagram will be the opposite of yours.
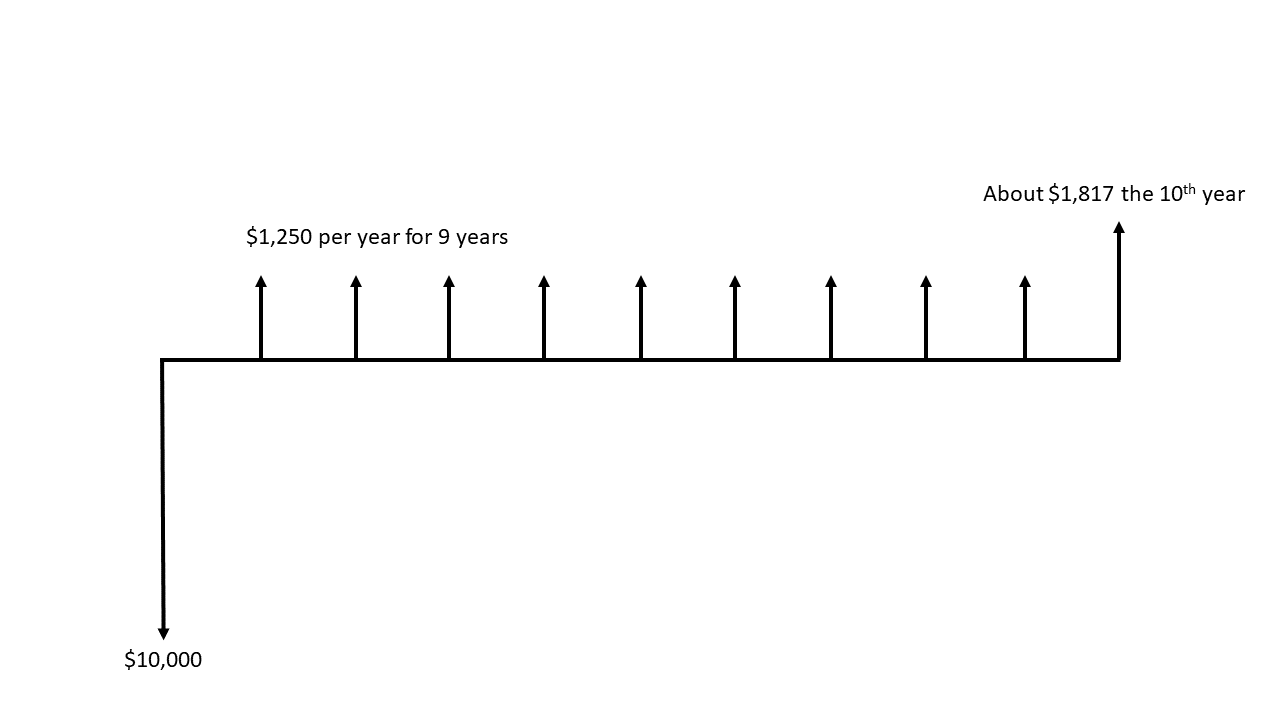
These are simple cash flow diagrams. It is possible to represent complicated funding and revenue schemes with cash flow diagrams. We already discussed loans and savings accounts in the previous section. Three additional basic tools4 are used to finance projects: mortgages, bonds, and sinking funds. We will discuss each of these in turn.
4.4.3.1 Mortgages
A mortgage is a loan for the purchase of property that uses the property as collateral. A bank or other investor gives a person or a company money to purchase a property (often a home). The property owner then makes regular payments to the bank to pay back the principal on the loan as well as the accumulated interest. If the owner is unable to make these payments, the bank can cancel the mortgage, seize the property, and resell the property to cover its losses. In the commercial world, mortgages allow the financing of large projects that would otherwise be too expensive to fund. They also allow people to buy houses that cost many times their annual salary and build real wealth.
Interest rates change for many reasons. Typically when the economy is doing well, interest rates rise to encourage people with money to invest it. When the economy is hurting, interest rates fall to encourage people to innovate or restructure their debts.5 So it might happen at some point that a person could obtain a loan for an existing mortgage at a lower rate than they are currently paying. By refinancing, they can take out a second loan at a lower interest rate to pay off the original loan, and then continue paying off the new loan at the lower interest rate.
Question
Is a mortgage a simple loan or a compound interest loan?
In a mortgage, you don’t accrue interest, because you pay all the accrued interest in each period. Because you don’t pay “interest on interest”, shouldn’t a mortgage be considered simple loan?
You might think so initially, but the answer is no. In a simple loan, the lender pays interest on the principal, but you don’t pay back the principal on a scheduled time-table like a mortgage. This is where the difference lies.
In a mortgage, the borrower pays the accrued interest and a fraction of the principal. Each mortgage payment is of equal value, with the initial payments mostly paying interest, and the last payments mostly paying principal. If you pay additional principal at the beginning of the loan, it has a compounding effect on the remaining mortgage payments. In each subsequent mortgage payment, more of your mortgage payment goes towards the principal and less goes towards interest. This has a compounding effect on the amount of interest you pay through the life of the loan.
For example, with a simple loan where you only pay interest and pay the principal at the end of the loan, you would pay twice as much interest if the length of the loan doubled (e.g. 15 years to 30 years). In a mortgage, you would pay more than 2.2 times as much interest with a 30-year mortgage compared to a 15-year mortgage with a 6% interest rate. (Note the relative difference in total interest between the mortgages depends on the interest rate and the length of the initial loan). See the Home loan homework question.
Short story, use the compound interest equations to calculate the monthly payments, present value, or future value of a mortgage.
In fact, if you had the option to either pay down the principal in your mortgage or save the money in an bank account with the same interest rate, compounding frequency, and time horizon, you would save the same amount of money either way. Thus, making additional early payments on a mortgage can save you substantial amounts of money later on.
4.4.3.2 Bonds
Bonds are agreements where a private investor loans a sum of money to a company or a government, and the company pays this principal (called the face value) back at the end date of the bond. The borrower also agrees to make regular interest payments (called the coupon) to the bond holder until the bond’s end date.
Because the interest doesn’t accrue, and the face value is not paid until the end date of the bond, a bond is a form of a simple interest loan. You can calculate the total interest you need to pay on a bond using Equation 4.11.
It is possible for a bondholder to sell their bonds to other investors. This might be advantageous if interest rates increase, allowing an investor to buy bonds with higher payments. On the market, bonds can be described in two terms:
- value: the present worth of all future bond payments, including the payback amount. This will not necessarily be the sale price of the bond, because other bonds on the market might have more or less of their value in the interest payments.
- yield: the ratio of the annual interest payment to the bond price.
If an institution fails to pay its bondholders, the institution is considered in default, and the bondholders can force the institution into bankruptcy. Institutions that are more likely to default therefore must pay higher interest rates to attract investors, and institutions that are unlikely to default (like the United States Treasury) pay very low rates compared to their peers.
Bonds are an especially important way for governments to raise money from many investors. In the case of a mortgage, most banks have the capital necessary to issue loans for houses (even many houses). But it would be very difficult for a bank to gather enough money to make a loan that would build an interstate freeway. It would also be impossible for a bank to repossess the interstate if the Department of Transportation couldn’t pay back the loan. Hence the importance of trust.
A special kind of bond can happen when the infrastructure will generate revenue, such as for a toll road. In this case, the investors might buy a portion of the tolls collected from the facility in exchange for a lower interest payment or a lower payback amount.
Think
In revenue-financed bonds for toll roads, the forecasts of traffic engineers play a critical role in helping investors to set the terms of the bond. What might happen if the traffic forecasts are wrong?
Think
If municipalities don’t have enough money to pay for the infrastructure in the first place, how can they afford to pay off the face value of the bond at the end of the bond?
Municipalities use money from taxes to pay for the interest and put money aside to pay for the face value in a sinking fund (See Section 4.4.3.3). Putting aside money to pay for interests and bond principal is called debt services (MRSC 2024). The US government used 17% of the federal budget on debt services in 2024 (Treasury 2024).
4.4.3.3 Sinking Funds
Sinking funds are used to put aside money for a future expense. A company often creates a sinking fund for the payment of bonds, or for the eventual repayment of a large debt. Sinking funds can also be a powerful tool in individual retirement. By setting aside a set amount of money every month, a person can eventually create a large sum of money that will gain enough interest to pay their living expenses and allow them to retire. Letting time and interest work for you and investing now is much efficient than trying to save in the future.
The United States Highway Trust Fund was originally created as a sinking fund. Gasoline tax revenues would fill the fund, and transportation infrastructure projects would be paid from the interest on the fund. Unfortunately, the federal gasoline tax was set at a particular level (18.4 cents per gallon in 1993) and was not indexed to inflation or any other metric. Politicians in both major parties have resisted raising the gasoline tax in the last 30 years. This means that the purchasing power of the gas tax revenues have eroded from inflation even while increasing fuel efficiency lowers revenue per vehicle mile and construction prices have increased. The Highway Trust Fund now operates more like a dedicated budget, with Congress periodically needing to appropriate funds from other sources to pay for highway and transit infrastructure.
Think
Is the current state of the Highway Trust Fund sustainable? Is that a problem? If not, what would be needed to make it sustainable?
4.5 Equivalence
Equation 4.14 introduced the idea that the future value of money \(F\) is different than its present value after interest and time have been considered. We can turn this equation around to get the present value of future money as well:
\[ P=F(1+i)^{-n} \tag{4.26}\]
Now we can convert Future cash flows to the present. It should be noted that \(i\) can also be referred to as the discount rate, because it represents the discount that needs to be applied to money from the future when converting it to present money. This means that money from the future is valued less than money in the present.
This is powerful because it allows us to translate complex cash flows defined with different interest rates and time frames into an equivalent present value.
Different people and organizations value time value of money differently. Also, the same people and organizations, will value time value of money depending on the investment. For example, investors will expect higher returns on more risky investments.
As we have talked previously, the reason to invest in a projects is to receive a return of benefits. If you are a developer, you want to build something that will generate income justifying the cost of construction. If you are building public infrastructure, the benefits might not be economic (although they could be). We will now consider definition of returns that considers the time value of money.
To do so, we need to be more precise about the timing of the returns. We will use the definition of returns in Equation 4.27.
\[ \mathrm{Returns}_t = \mathrm{Asset\ Sale}_t + \mathrm{Revenue}_t \\ - \mathrm{Asset\ Purchase}_t - \mathrm{Expenses}_t -\mathrm{Interest}_t - \mathrm{Taxes}_t \tag{4.27}\]
Where
- \(R_t\): returns in year \(t\)
- \(Asset\ Sale_t\): The sale price of an asset
- \(Asset\ Purchase_t\): the purchase of the asset, this includes outright purchase of the asset, downpayment of principal, the portion of your mortgage that goes towards paying principal, and the final loan payoff if you sell your asset before the end of the loan.
When Equation 4.27 is calculated over the liftime of the assets, then \(\Delta(\mathrm{Asset\ Value} = \mathrm{Asset\ sale}-\mathrm{Asset\ Purchase}\), and we obtain the earilier Returns equation in Equation 4.1.
Because we often purchase an asset with a loan, we can separate the Asset purchase into the Loan down payment, Loan payment of principal, and the Loan payoff. The Loan payment includes both the payment of both principal and interest. This yields Equation 4.28:
\[ \mathrm{Returns}_t = \mathrm{Asset\ Sale}_t + \mathrm{Revenue}_t \\ - \mathrm{Expenses}_t - \mathrm{Taxes}_t \\ - \mathrm{Loan\ down\ payment}_t -\mathrm{Loan\ payment}_t - \mathrm{Loan\ payoff}_t \tag{4.28}\]
We will use these equations for calculating yearly returns in the next section.
4.5.1 Net Present Value
Using the time-value of money equations we learned in the previous section, and with a discount rate, we can find the net present value (NPV) of a project, or the current value of all future cash flows. If the NPV is positive, then the project will result in a positive return on investment. If it is negative, then the project will lose money over its life. The equation for the NPV is \[ NPV=\sum_{t=0}^{n} \frac{R_t}{(1+i)^t} \tag{4.29}\]
where * \(R_t\): net returns at time \(t\) * \(i\): interest / discount rate * \(t\): period of time
Note that the \(1/(1+i)^t\) is the same as \(P/F(i, t)\), or the equivalence function to bring a future value to the present.
How much the project gains or loses is an important consideration beyond the binary win / lose scenario: every investment has an opportunity cost. If I invest in one project, I cannot invest in another. So we need some way to determine what the return is. I should look for investments that maximize my return.
4.5.2 Setting the MARR
This brings up the question of what discount rate should be used in NPV calculations, and how this discount rate is determined. Recall that the discount rate has to account for:
- Risk: when you lend money, it might not always come back
- Inflation: money will be worth less in the future
- Opportunity Cost: you might be able to do something else with your money
In this way, the discount rate represents a Mimumum Acceptable Rate of Return (MARR). If a project’s returns are lower than the combined risk, inflation, and opportunity cost, then it is not a smart investment.
Every actor in an economy including business owners, developers, investors, and individuals will have their own MARR when evaluating a project. Let’s unbundle the three elements of the discount rate: \[ F = P*(1 + \text{risk})^n(1 + \text{inf} )^n(1 + \text{opp.cost})^n \]
Because each of these rates individually is small (somewhere less than 10%), we can simplify this to a single rate that includes all three elements, \[ F \approx P(1 + \text{risk} + \text{inf} + \text{opp.cost})^n \tag{4.30}\]
We can also make this assertion because each element of \(i\) cannot really be definitively known. Having said that, there are reference points that can be used for these values. Governments regularly publish inflation statistics; using the long-run or recent short-run average inflation rate could be a good benchmark depending on the application. Further, government bonds for stable countries are seen as relatively risk-free investments; at the very least, the MARR for an investor should exceed the interest paid on those bonds. Finally, the market can show what investors are currently paying for bonds of equivalent risk.
When a company is considering the MARR for its own projects, it considers the cost of raising capital. If the return on the investment is less than the cost of raising capital, the company will lose money.
As mentioned in Section 4.4.3, businesses raise capital through debt or equity financing. Often they will use a combination of both debt and equity financing. One reason companies raise money from both methods is because the costs of debt financing tends to increase as they comprise a higher fraction of the cost of the project. For example, lenders may consider a project risky that is debt financed for more than 50% of the costs.
The cost of capital when it is a combined sources of capital is called the weighted average cost of capital (WACC). The WACC is calculated in Equation 4.31.[Blank and Tarquin (2023a)]
\[ \mathrm{WACC}= (\mathrm{equity\ fraction})\times{(\mathrm{cost\ of\ equity\ capital})}+ (\mathrm{debt\ fraction})\times{(\mathrm{cost\ of\ debt\ capital})}\\ \tag{4.31}\]
Where the cost of debt capital is the interest rate on the debt. The cost of the equity is the expected rate of return of its company shares. By selling the shares, the company will not realized those returns, in other words, the equity costs is the opportunity cost of choosing to sell their equity.
A company’s MARR should therefore be at least the value of the WACC. Of course, a company will not pursue every project with returns greater than its WACC, because doing so might expose the company to lots of risk, which would raise the MARR for investors that might buy the company’s stock. A company might set its MARR differently for projects of different sizes, with differing ranking and selecting criteria.
For an individual, the MARR should reflect inflation, risk, and the time that could be spent working on something else. In a very real sense, MARR is subjective.
4.6 Internal Rate of Return
In Equation 4.2 we looked at how to compute the return on investment for a one-year investement. But most projects in civil engineering and construction are not one-year investments. Often, people will build expensive infrastructure hoping to earn revenues over many years. It would be nice to know what the average rate of return on a project is when the costs and revenues occur in different years. It is also important to consider that because of the time value of money, dollar in different years have different values. In this section, we will define and use a measure called the internal rate of return (IRR) that accounts for these things.
The IRR of an investment is defined as the interest rate that makes the net present value zero. Or put another way, it is the interest rate at which all future earnings are equal to the investment.
Consider a simple project with only a present investment value \(P\) and future returns value \(F\), as in Equation 4.14. The net present value of this project is \(NPV = -P + F*P/F(i, n)\) Let’s solve this equation for the discount rate \(i\) when the NPV is zero: \[\begin{align} NPV &= -P + F*P/F(i, n) \\ 0 &= -P + \frac{F}{(1 + i)^n} \\ P &= \frac{F}{(1 + i)^n} \\ \frac{F}{P} &= ( 1 + i)^n \\ \left(\frac{F}{P}\right)^{1/n} &= (1+i) \end{align}\] which reduces to \[ i = \left(\frac{F}{P}\right)^{1/n} - 1 \tag{4.32}\] So, there is one particular value of \(i\) that effectively sets the future returns equal to the present investment value, with \((F/P)^{-n}= 1\). This is the IRR.
Why is this value a rate of return, or a measure of return at all? Conceptually, it shows how much our initial investment grew over the course of our project, just like ROI is supposed to. But it works mathematically as well. We can add \(P/P\) and substract \(P/P\) (effectively adding 0) inside the parentheses, \[\begin{align} i&= \left(\frac{F}{P} - \frac{P}{P}+\frac{P}{P}\right)^{1/n} - 1 \\ &= \left(\frac{F-P}{P}+1 \right)^{1/n} - 1 \\ \end{align}\] Now, let’s consider that our traditional ROI equation in Equation 4.2 can be redefined as \(ROI = \frac{F-P}{P}\) with the returns from our investment divided over the amount of the investment. This the value we have inside our rearranged Equation 4.14! \[ i= \left(ROI +1 \right)^{1/n} - 1 \tag{4.33}\] Effectively, \(i\) in this case is an annualized ROI (Beattie 2024), with each year’s returns adjusted for inflation, risk, and opportunity cost.
IRR
You invest \(P\) into a savings account which yields a future value \(F\) after \(n\) years. The interest rate, \(i\), on the savings account is compounded yearly. Which of the following terms best describes \(i\)?
- Interest rate
- APY
- Annualized ROI
- Internal rate of return
This is a tricky question. In this simple case \(i\) can correctly be defined by each of the terms. All of these values are the same in definition. However, only IRR is available when the cash flows get complex. Please note that sometimes the IRR is referred to as the rate of return or the ROI. For exams, and in this textbook, we will refer to the IRR as the IRR. The ROI will be used to refer to the return on investment over a period of time.
The benefit of IRR is that once we have calculated the NPV of a project — no matter how complex its costs and earnings — we now have a tool to immediately calculate the implied return from this project, and we don’t have to even know what the interest rate is, because it’s something we calculate in the problem.
For example, what if you make an investment \(P\), in order to receive an annuity of \(A\) over the next \(n\) years. What is the rate of return of your investment? We can use Equation 4.20 \[\begin{align*} P &= A*\frac{(1 + i)^n -1}{i (1 + i)^n} \end{align*}\] Unfortunately, Equation 4.20 is too complex to re-arrange to solve for \(i\) by algebra. Fortunately, there is another way to solve for \(i\). We can construct the net present value of this cash flow, \[ NPV = -P + A * P/A(i, n) \] and find the interest rate that sets \(NPV = 0\). We can do this by brute force — trying values until we get the right one — or by using optimization software included in spreadsheets or other programs.
We will now provide several examples on how to solve for the IRR with more complex cash flows.
IRR Example with Factor Tables
Margot decides to pursue a PhD degree in Civil Engineering while she is working. She pays upfront $30,000 for her tuition and books at the start of her program. She then earns an additional $5,000 per year for the next 5 years while a research assistant.7 In year 5, she successfully led a team to design an earthquake resistant bridge that was a direct outcome of her thesis. The President of her engineering firm gives her a bonus of $14,746.84. What is the IRR of her doctoral investment after 5 years? Is it 6%, 8%, or 10%?
To solve for IRR, we need to find the interest rate, \(i\) that yields an NPV = 0. Let’s draw a cash flow diagram of this problem to help us set up the NPV equation.
The upfront tuition and books are a present value \(P\), the RA wages are an annuity \(A\), and the bonus she gets is a future value \(F\). So the NPV equation we need to solve is
\[0 = P + A *P/A(5, i) + F * P/F(5, i)\] Let’s set up a table that will calculate this equation for different values of \(i\). The final column, NPV is the equation above using the factors on that row. You can see that with a discount rate of 8%, the NPV is effectively zero, meaning that the IRR is very close to 8%.
| i | P | A | A/P | FV | F/P | NPV |
|---|---|---|---|---|---|---|
| 0.06 | -30000 | 5000 | 4.2124 | 14746.84 | 0.7473 | 2081.5156 |
| 0.08 | -30000 | 5000 | 3.9927 | 14746.84 | 0.6806 | 0.0017 |
| 0.10 | -30000 | 5000 | 3.7908 | 14746.84 | 0.6209 | -1889.4387 |
We can also solve this more precisely using a numerical solver. In this case, I can use the R optim function which works like goal seek.
# calculate NPV for different values of i
irr_example_fn <- function(i){
NPV <- -30000 + atop(5000, i, 5) + ftop(14746.84, i, 5)
abs(NPV) # find smallest NPV, positive or negative
}
# find value of i that makes this function 0, within bounds
o <- optim(0.06, irr_example_fn, method = "Brent", lower = 0, upper = 1)
o$par # This is the value of i that makes NPV 0[1] 0.08000002Sure is close to 8%.
And finally, here is a video showing how to solve the problem with goal seek:
The IRR is the rate of return of an investment. However, there are weaknesses. First, when solving for the IRR, sometimes your solver will not find the solution, which can also depend on your starting location. Second, under certain circumstances, the IRR for a given cash flow is not unique; e.g., a complicated cash flow might show a zero NPV at 2% and at 9%.
4.7 Project Assessment
In the previous section on returns, we looked at project evaluation largely as a binary consideration: is the IRR greater than the MARR? Or, is the NPV positive?
It is rare to only have one course of action in an investment. More typically, there are multiple different strategies or alternatives that you need to weigh against each other. In this section, we will discuss how to comparatively evaluate projects with different timelines, discount rates, and other variables.
4.7.1 NPV and IRR
NPV and IRR are both important methods to compare projects. These measures can sometimes identify different preferred alternatives, however this is not always the case as shown in the following example.
Think
Should you use NPV or IRR to prioritize projects?
That depends. If the projects are mutually exclusive (e.g. there are different alternatives for the same project, and you only can pick one alternative), then it is recommended to select the project with the largest net present value. (Blank and Tarquin 2023b).
If the projects are independent from one another (e.g doing project A doesn’t stop you from doing project B) then a good approach is to rank the projects by the rate of return. (Blank and Tarquin 2023c). First do the project with the best rate of return, if you have sufficient remaining funds, then do the project with the next best rate of return, and so on. Other methods to rank independent projects projects given budget constraints are discussed in (Blank and Tarquin 2023c).
4.7.2 Benefit Cost Analysis
Private projects often will use the rate of return or the net present value to select between alternatives. This evaluates how much returns will return to the firm. For governmental or charitable non-profit organizations may want to pick a project that provides the most benefits to the public rather than the highest returns to the company. Benefit cost analyses (BCA) balances the benefits and costs of a project incurred to the public. Benefit cost analysis is also referred to as Cost-Benefit Analysis (CBA). BCA uses two different metrics, net benefits and benefit cost ratio (B/C). (FHWA 2020)
4.7.2.1 Net Benefits
The net benefits are similar to the net present value, and are calculated as the present value of the benefits subtracted by the net costs, as shown in the equation Equation 4.34.
\[ \mathrm{Net\ Benefit}s=\mathrm{Present\ Value\ of\ Benefits} - \mathrm{Present\ Value\ of\ Costs} \] Expressed mathematically as:
\[ \mathrm{Net\ Benefits}=\sum_{t=0}^{n} \frac{B_t}{(1+i)^t}-\sum_{t=0}^{n} \frac{C_t}{(1+i)^t} \tag{4.34}\]
Where
- \(B_t\): Benefits at time \(t\)
- \(C_t\): Costs at time \(t\)
- \(i\): discount rate
- \(t\): period of time
Benefits could include revenue, (such as tolls for a highway project) or funds saved (such as lower energy costs). Costs include the capital costs to build the project, operating and maintenance costs, and interest payments. With large multi-year infrastructure projects with long term impacts on the public, we account for the time value of money when calculating net benefits as shown in Equation 4.34.
The benefit and the costs can be external to the organization building of funding the project. For example, the Federal Highway Administration (FHWA) lists the following as potential benefits and costs that can be included in an analysis of a highway project.(FHWA 2020)
- Travel time (and the reliability of travel time)
- Crashes
- Fuel use
- Vehicle operating costs
- Emissions/air quality
- Agency efficiency
In addition, the benefits could include lower costs due less risk of damage from a natural disaster (for a new flood levee or culvert). To include these external costs in a benefit-cost analysis, we need to be able to monetize these benefits and costs. As expected, many of the external or preventative benefits and costs are difficult to monetize. Economists have developed prices for some of these intangible costs, including the value of increased safety, by putting a value on human life.
Think
Is it ethical to put a price on a human life when evaluating infrastructure projects?
Imagine what would happen if we planned all projects to try to eliminate all risks to zero. Despite our best intentions, we can never completely reduce the risks of dying due to building failure, traffic crashes, or exposure to harmful pollutants. Even if we did, we would not accept the costs of such actions. e.g. if everyone traveled at a max speed of 20 mph, we would likely save lives from traffic accidents, but the cost of the extra travel time would be too high.
Federal agencies such as the Federal Highway Administration (FHWA), and the US Environmental Protection Agency (US EPA) use the value of the statistical life (VSL) in benefit cost analyses of projects, programs, and rule makings. By using the VSL, we can monetize the benefits of increased safety or decreased exposure to pollution, to then choose an acceptable amount of risk by balancing both cost and safety.
The US EPA describes VSL as (EPA 2014)
The EPA does not place a dollar value on individual lives. Rather, when conducting a benefit-cost analysis of new environmental policies, the Agency uses estimates of how much people are willing to pay for small reductions in their risks of dying from adverse health conditions that may be caused by environmental pollution.
For example, the US EPA used a VSL of $11 million dollars in a cost-benefit analysis of a proposed rule to lower emissions from heavy-duty trucks. (US EPA 2022)
You can quantify the benefits from lives saved due to increased safety or less pollution exposure in terms of reduced risk from a baseline condition. The risk for a certain negative outcome (such as death or injury) is calculated using Equation 4.35.
\[ \mathrm{Risk} = \mathrm{number\ of\ people}\times\mathrm{probability}\times\mathrm{impact} \tag{4.35}\]
Where
- number of people: number of people potentially impacted by the negative outcome
- probability: probability of the negative outcome occurring to each person
- impact: the cost of the negative outcome occurring to a person
The benefit is then calculated as:
\[ \mathrm{Benefit}_{alternative} = \mathrm{Risk}_{baseline}-\mathrm{Risk}_{alternative} \tag{4.36}\]
4.7.2.2 Benefit Cost ratio
The second metric we use in benefit cost analyses is the benefit to cost ratio, \(\ (B/C)\). The benefit cost ratio is similar to the ROI, in that it considers the benefits compared to the costs.
\[ \mathrm{Benefit\ Cost\ Ratio} (B/C)=\frac{\mathrm{Present\ Value\ of\ Benefits}}{\mathrm{Present\ Value\ of\ Costs}} \tag{4.37}\]
If the ratio \(B/C > 1\), then the project is feasible. If the \(B/C <1\) the costs outweigh the benefits and the project is not recommended. If a project has a higher \(B/C\) ratio than another project, it could be more attractive than other alternatives.
Do you use the Net benefits or use the B/C ratio in prioritizing projects? Both provide useful information, however you may want to consider using these metrics the same way we recommend using NPV and IRR. If you projects are mutually exclusive, you should pick the alternative that maximizes the net benefits. When you are evaluating many independent projects, you may want to prioritize the projects with the highest B/C ratio.
Note
In all of the examples we have covered, the benefits and costs of the projects were taken as given. In the real-world, almost all of the revenues and costs can only be estimated (save perhaps a loan payment which may be known). You should consider how confident you are in those estimates when conducting a financial or benefit cost analysis of a project. One way to do that is using an evaluation matrix discussed in the next section.
4.7.3 Evaluation Matrices
While benefit cost analyses are useful, decision makers often consider additional factors regarding large infrastructure projects. Large public projects have benefits and costs that occur on a wide spectrum of different dimensions. It can be difficult or impossible to monetize all benefits and costs in a BCA. Also, in a simple cost benefit analysis, we don’t consider social justice, or the equal sharing of costs and benefits (e.g. Who is benefiting the most from this project?, Who is most adversely impacted by this project?). We can use the triple-bottom line discussed in Chapter1 as a guide in making decisions, but within each of these dimensions can be multiple different variables that require consideration. A common method to put these variables into one decision making framework is by using an alternatives evaluation matrix.8 To create this matrix:
- Decide which elements of a project, in terms of benefits and costs or other outcomes need to be considered. This may involve public input.
- Develop a scoring system \(b\) for each element. This is often a score of 1 to 5 or a grade of A to F or some other categorical measure.
- Develop weights \(w\) for each element, again with public input.
- Calculate the weighted score of each alternative as \(S_a= \sum_e w_e*b_{ea}\) where \(S\) is the total weighted score for the alternative, \(w_e\) is the weight of each element \(e\), and \(b\) is the score of within that element for each alternative.
Figure 4.6 shows a project evaluation matrix from the Utah Transit Authority’s Point of the Mountain alternatives analysis. UTA has developed five different alternatives to provide high-frequency transit to Lehi and the site currently occupied by the Utah State Prison, using different combinations of light rail extensions and new BRT service. These five alternatives are each evaluated on a five-point scale applied to 19 different measures grouped into five general categories:
- Transit System Performance
- Access and Mobility
- Land Use
- Cost and Constructability
- Natural and Built Environment
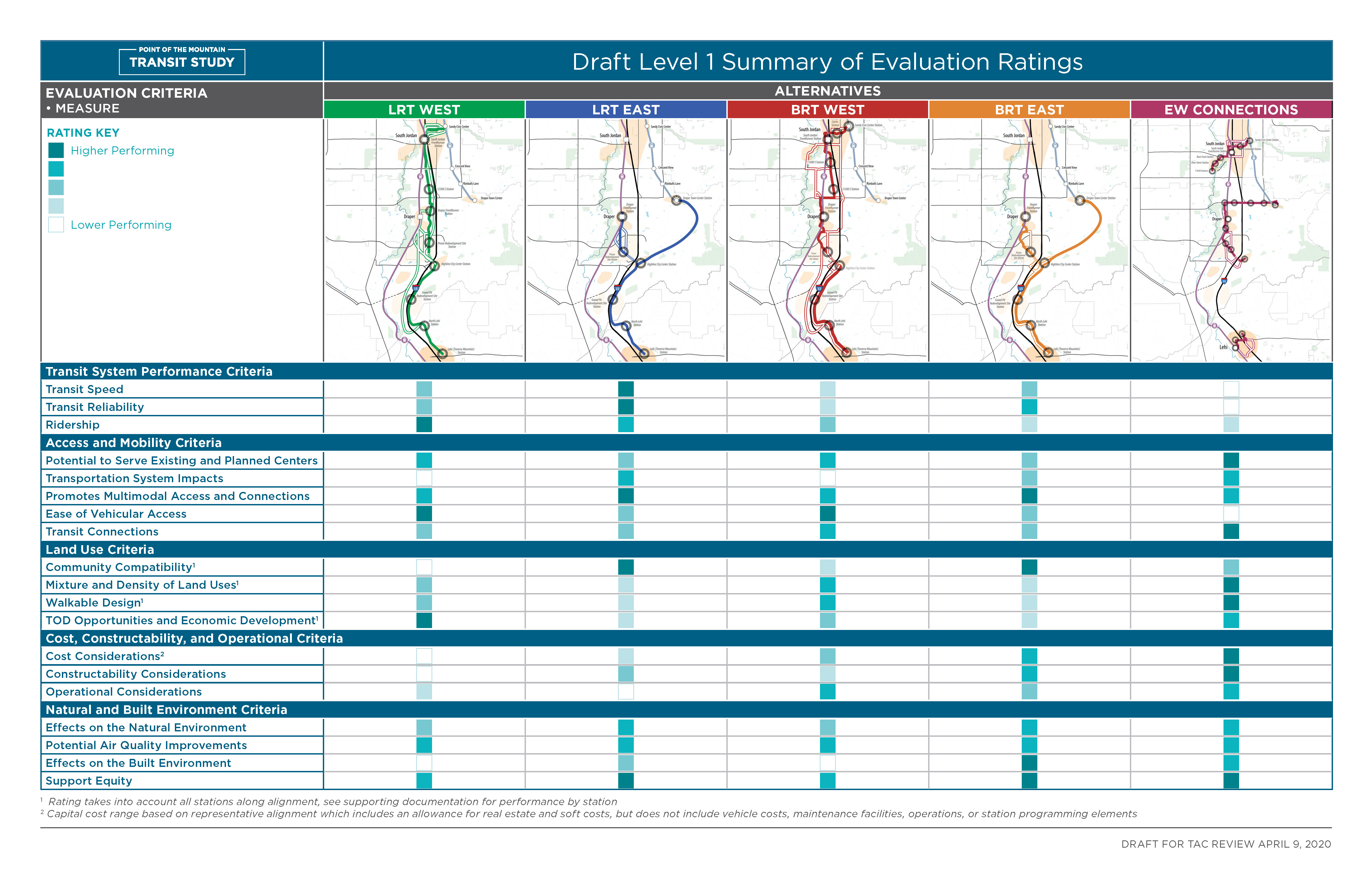
You can see in the figure how each alternative generally performs. The EW Connections alternative scores the best in terms of cost, but it performs poorly in terms of transit speed and reliability. The LRT East and BRT East alternatives have similar profiles, but the BRT option trades a bit of speed and reliability for a lower cost.
When conducted in this way, a number of different factors besides pure cost and benefit are allowed to contribute to the overall project evaluation. There is also a risk, however, that this method can become purely subjective if care is not taken to avoid pre-determining the outcome.
Unit Summary
The time value of money means that $100 now is worth more than $100 in the future because of risk, inflation, and opportunity cost. This makes interest a powerful tool in funding projects with things like mortgages, bonds, sinking funds, and toll-based financing. Cash flow diagrams provide an easy way to organize the cash flows of projects over long periods of time. Using equivalence equations for present value, future value, and annuities allows us to calculate the net present value (NPV) from a cash flow diagram, which determines if a project will be profitable. We must pick an appropriate discount rate in order to correctly calculate the present value of future cash flows and to meet our minimum acceptable rate of return (MARR). We can also calculate the internal rate of return for complex cash flows from infrastructure projects.
Ultimately, though, decisions cannot be based on finances alone. They must also incorporate costs and benefits that do not have monetary values, either by determining a value for them or by using a suitable weighting system for different categories. Sustainable infrastructure can only be achieved if all of these factors are considered.
- annuity (A): a series of equal payments made over time
- bond: financial tool in which an investor loans a sum of money to an organization, and the borrower pays annual interest on the loan until the end date of the bond, at which point they pay back the face value
- book value: the initial cost of an asset minus total depreciation
- cash flow diagram: a graphical display of cash inflows and outflows over time
- compounding frequency: the frequency with which the interest on a loan is re-applied to the principal
- depreciation: the value of an asset lost over time due to use, found using methods like straight-line or MACRS
- discount rate: an interest rate used to determine the present value of a future cash flow
- external rate of return (ERR): the discount rate at which the present value of investments is equal to the future value of earnings
- factor tables: tool for converting cash flows (PV,FV,A,G) into equivalent time values of money
- future value (FV): the equivalent future worth of a sum of money or series of payments
- gradient (G): a series of equally increasing payments made over time
- inflation: the increase in price for goods and services over time
- interest: money paid by a borrower to a lender in exchange for the use of their money
- internal rate of return (IRR): the discount rate that makes the NPV of a project equal to zero
- minimum acceptable rate of return (MARR): the lowest rate of return that a project must earn in order to be worthwhile to an investor
- mortgage: a loan to purchase property using that property as collateral, repaid in regular periodic amounts
- net present value (NPV): the equivalent present value of all future cash flows
- opportunity cost: the potential benefits that are lost when one option is chosen over another
- present value (PV): the present worth of a sum of money or series of payments
- project assessment: comparing projects using methods such as NPV, ROI, B/C, and incremental B/C
- project evaluation matrix: a tool to compare several alternatives across an array of quantitative and qualitative criteria
- refinancing: taking out a second loan at a lower interest rate to pay off an original mortgage
- return on investment (ROI): the ratio of the equivalent annual earnings to the total investment
- risk: the chance that an investment will be less profitable than expected, including the possibility of losing some or all of the original money
- sinking funds: a fund where smaller amounts of money are put aside regularly to pay for some larger expense in the future
- time value of money: the concept that money now is worth more than the same amount of money later because of risk, inflation, and opportunity cost
- toll-based financing: loans to build infrastructure are repaid using future revenue from tolls and service fees
- zoning: laws that specify what types of projects can be built in certain areas
Homework
Problem Set: Investments and ROI
HW 1: Investments and Returns
An engineering firm is considering investing in a renewable energy project that costs $750,000. Over the next 5 years, the project is expected to generate annual revenue of $200,000 per year. However, the operating and maintenance costs for the project cost $50,000 per year.
- Calculate the returns for one year.
- Calculate one-year ROI.
- Calculate the returns for five years.
- Calculate the five-year ROI
HW 2: Rental Property
You purchase a rental property in Provo for $600,000 at the end of 2022. For all 12 months of 2023, you rent out the home for $3,000/month. You pay an average of $500 dollars/month in property taxes and $400 dollars/month in utilities. You also pay for $30,000 of repairs during the year. At the end of 2023, you sell the investment property for $650,000.
- What are your returns?
- What is your ROI?
HW 3: Excavation
You own an excavation company. You are considering buying an additional excavator for $125,000 and hiring someone to operate it. With the excavator, you estimate your additional annual revenue and expenses to be:
| Revenue | Fuel | Maintenance | Insurance | Operator Wages |
|---|---|---|---|---|
| $120,000 | $10,000 | $10,000 | $15,000 | $50,000 |
- Considering just the revenue and costs above, what are your first-year returns?
- What is the simple pay back period for the excavator? Provide the answer in years and months.
- What is the 1-year return on investment (ROI)?
HW 4: Excavation Loan
Now assume that you take out an equipment loan to pay for the excavator. In order to qualify for a reduced interest rate of 6%, you need to make a 20% down payment.
- What are your returns in the first year?
- What is your 1-year ROI?
- Compared with the previous problem, should you take out a loan for the excavator?
HW 5: Investments, Returns, and ROI
You are working as a project manager for a civil engineering firm, overseeing the development of a large residential subdivision. The project involves constructing 200 homes, and each home will require an investment of $500,000 for land acquisition, materials, labor, and infrastructure (including roads, water systems, and utilities). After completing the project, you estimate that the company will generate an average profit of $100,000 per home due to favorable market conditions.
- What is the total investment required for the entire subdivision?
- What will be the total returns from the project?
- Calculate the project’s ROI over the time period of the project.
Problem Set: Taxes and Depreciation
HW 6: Book values
A company purchases a bulldozer for $200,000 and expects a salvage value of $30,000 at the end of the 7-year recovery period. Using both the straight-line and declining balance depreciation methods, calculate the depreciation and book value of the asset at the end of each year of the recovery period. Which method will have a lower tax burden in year 6, all else equal?
HW 7: Excavator Depreciation
Reconsider the excavator problem in HW 4 (where you took out the loan), but include the depreciation of your excavator and the taxes you need to pay to operate. Assume that the excavator has a 10-year lifetime with a salvage value of $10,000, and use straight-line depreciation. Use a 33% effective tax rate.
- What is the annual depreciation value?
- Re-calculate the annual returns and the simple payback period.
- Re-calculate the 1-year ROI considering the excavator depreciation.
HW 8: A fleet of excavators
Your company purchased an excavator in 2019, 2021, and 2022; each excavator cost $125,000. What is the total book value of all of your excavators in 2025 by the declining balance method? Construction equipment has a five-year recovery period.
Problem Set: Time Value of Money
HW 9: Time value F from P
A $5,000 investment earns 6% per year. What will be the value of this investment in 15 years if:
- The investment is compounded annually?
- The investment is compounded monthly?
HW 10: Time value P from F
When you were born, your grandparents purchased shares in a mutual fund paying 8% interest per year compounded annually, hoping that this investment would pay for your college tuition (lucky!). Assuming you are at BYU for eight semesters beginning when you are 19, how much should they have invested?
Assumptions
This problem requires you to make assumptions. There is not a single correct answer as different assumptions can be valid. Document your assumptions, and show your work.
HW 11: Comparing F
Sarah and Brian both invest in a retirement fund. Sarah invests $15,000 at age 30, while Brian invests $40,000 at age 50. Both accounts earn 8% interest per year, compounded annually.
- How much each will they each have at age 65?
- How much would Brian need to invest at age 50 to have the same amount as Sarah by age 65?
HW 12: Simple Uncle Shark
You borrow $20,000 from your uncle to help pay for four years of college (because your grandparents didn’t buy you a mutual fund…). You agree to a simple interest loan with an annual interest rate of 6% on the remaining principal owed at the end of each year. Each summer in college (before the end of the year) you work in his company and earn $4,000 which you use to pay down the loan principal. You then pay the entire loan off with your first out-of college paycheck at the beginning of year 5.
- How much total interest do you pay your uncle over the course of the loan?
- What is the payoff amount?
Include a table like the one below tracking your loan (you might find it helpful to have other columns as well).
| Year | Principal | Interest | Loan Balance (End of Year) |
|---|---|---|---|
| 1 | |||
| 2 | |||
| 3 | |||
| 4 |
HW 13: Compound Uncle Shark
Repeat the previous problem, but the loan is compounded annually. That is, the interest is calculated on the entire remaining balance rather than just the principal amount.
Problem Set: Annuities and Gradients
Tip
I recommend solving the problems in this problem set primarily with the factor tables in Appendix A, so you can get used to using them.
HW 14: Car Maintenance
Frank’s parents give him an old car that will last for 10 more years as long as he keeps repairing it. The car needs $500 worth of repairs the first year, and the maintenance costs will increase by $50 each year afterwards. How much money does Frank need to invest now at an 7% interest rate to pay for the next 10 years of maintenance on the car? Draw a cash flow diagram as part of your solution.
HW 15: Stock Market
You decide to invest in the US stock market, which has a historic return of 10% per year.
- How much would you need to invest each year to have $1,000,000 by age 65 if you started saving at age 25?
- How much would you need to invest each year to have $1,000,000 if you started at age 45?
HW 16: Depreciation in the present
You buy an excavator for $125,000 which has a 5-year recovery period and you expect to salvage the excavator for $25,000. What is the present value of all of the depreciation expenses if you use a straight line depreciation and assume a 6% discount rate? Include a cash flow diagram in your answer.
HW 17: Home Loan
You are buying a home for $430,000. Your 30-year mortgage has a 6.28% annual interest rate (compounded monthly) and requires a 20% down payment to secure.
- What is the monthly payment? Recall that \(i/k\) is the periodic interest rate.
- What is the total amount paid?
- For each month, calculate the amount of the payment that goes to interest and the amount that goes to principal. Create a plot showing these amounts over the entire course of the loan.
Help
Each month, your monthly payment includes part interest and part payment on your principal. If you pay all the interest that accrues each month, then any amount left over from your monthly payment will apply to the principal.
Set up a spreadsheet or computer program that calculates the amount paid to interest and to principal in each month of the 30-year mortgage. You should include example calculations and the top few rows of the table in your homework submission, but do not need to include the entire 360-line spreadsheet.
Also: if you have done this correctly, you will pay off the loan with your last payment.
HW 18: Home Loan Payoff
Consider the same information as the previous problem. What happens if you are able to pay an additional $200 each month?
- After how many years do you pay off the mortgage?
- How much total money do you pay?
- How much money do you save compared to the previous problem?
It is not necessary to include the graph again.
Problem Set: Equivalence
HW 19: Water lines
You are considering signing a contract to install water lines in a development project with a 5-year construction timeline. You expect your annual costs to be $400,000/year. The developer provides two options for your company’s payment.
- Option 1: The developer pays you $1,000,000 now (year 0), and $2,000,000 at the end of the project in 5 years.
- Option 2: The developer pays you $4,000,000 at the end of the project (in 5 years).
- Draw/graph a cash flow diagram of both options.
- Assume your company uses an annual discount rate of 12%. Calculate the present value for each of the payment options.
- Which is the preferred option using the net present value (NPV)?
- What other financial considerations are there that are not expressed in the NPV?
HW 20: Construction Company Present Value
You are a project manager for a construction company, and you are evaluating the potential investment in a new piece of heavy machinery. The initial cost of the machinery is $150,000. You expect this machinery to generate additional annual revenues of $45,000 for the next 5 years. At the end of the 5 years, the machinery will have a salvage value of $10,000. The company’s expected rate of return for their investments is 8%.
Calculate the Present Value of Future Revenues: Using the company’s expected rate of return of 8%, calculate the present value (P) of the additional annual revenues generated by the machinery over the next 5 years.
Calculate the Present Value of the Salvage Value: Calculate the present value (PV) of the salvage value of the machinery at the end of 5 years using the company’s required rate of return of 8%.
Calculate the Present Value of the Revenue and the Salvage Value: Combine the present value of the future revenues and the present value of the salvage value to determine the total present value (PV) of the revenues.
Determine the Net Present Value (NPV): Calculate the net present value (NPV) of the investment by subtracting the investment cost of the machinery from the present value of the revenue and salvage value calculated in question 3.
Evaluate the Investment Decision: Based on the NPV calculated in question 4, determine whether the company should proceed with the investment in the new machinery. Explain your reasoning.
HW 21: Air Conditioner
Alissa wants to install a new $9,000 air conditioning system. Because it is more energy efficient, the system would save $1,400 per year in electricity costs. Assume the air conditioner is installed in year 0, and savings begin with year 1.
- Without accounting for the time-value of money, what is the payback period?
- Assuming a discount rate of 7%, how long will it take for the air conditioning system to pay for itself? Assume the savings compound annually. Hint: Find the number of years where the net present value is 0. This problem can be solved in multiple ways: algebraically manipulating the formula to solve for \(n\), using goal seek to change \(n\), or plotting \(n\) vs. \(P\) in a table.
- Draw a cash flow diagram for each of the above parts. Make sure to label your axis and which diagram comes from which part.
HW 22: Bonds
Ben can buy a bond with a face value of $1,000 paying at 6% interest for five more years.
- Draw or graph a cash flow diagram for the bond.
- What is the present value of this bond if Ben’s personal discount rate is 5%?
- What is the present value at a 7% discount rate?
Note: to solve this problem, first draw the cash flow diagram for the bond. Then, determine the present value of the bonds using the different discount rates.
Problem Set: Internal Rate of Return
HW 23: Pavilion to rent
In 2019, you built a pavilion to rent for special events $15,000 and started operations in 2020. Each year you operate, you incur $100 in expenses. In 2020, you made no money because of the pandemic. But in 2021 you earned $1,000, and grew your business by an $1000 each year after (e.g. $2000 in 2022, $3000 in 2023, etc.). At the end of 2024, you sold the pavilion for $15,796.68.
- Draw a cash flow diagram of this project.
- Calculate the NPV of this investment given discount rates of 10%, 12%, and 18%.
- What is the IRR of this investment?
HW 24: Dock
You are considering building a new dock at a port. There are two options for the dock. Dock A is smaller, will cost $70 Million dollars, and will generate returns of $24 million dollars/year for the next 10 years. Dock B is larger. It will cost $170 Million dollars and generate $41 million dollars per year for the next 10 years.
- The port authority uses a discount rate of 8% to evaluate capital investments. What is the net present value of both options using the 8% discount rate?
- What is the internal rate of return for both options?
- Which dock would you recommend building? Why?
HW 25: Varying Incomes
In 2010, you made a $45,000 investment in new construction equipment, which you expected to generate revenue of $10,000 per year for the next ten years (2011 through 2020), on $3,000 of annual expenses. In reality, the revenue was the values in the actual column of the table below:
| year | expenses | expected | actual |
|---|---|---|---|
| 2011 | 3000 | 10000 | 7000 |
| 2012 | 3000 | 10000 | 10000 |
| 2013 | 3000 | 10000 | 13000 |
| 2014 | 3000 | 10000 | 18000 |
| 2015 | 3000 | 10000 | 11000 |
| 2016 | 3000 | 10000 | 2000 |
| 2017 | 3000 | 10000 | 8000 |
| 2018 | 3000 | 10000 | 12000 |
| 2019 | 3000 | 10000 | 7000 |
| 2020 | 3000 | 10000 | 14000 |
- Calculate the IRR for the expected project as well as what actually happened. Did things go better or worse than what you expected?
- Comment on what might happen in 2016 if this project was financed through a bank.
HW 26: The Big Kahuna
Difficult problem
This problem ties together almost everything you have learned in this unit. When you understand how to solve this problem, then you should be well-prepared for the test and to solve related problems in your career.
Advice to solve: clearly read the problem, and take each element in steps. Set up a spreadsheet to track each year’s returns, taxable income, and any values used to calculate those elements. As always, include example calculations in your homework response, alongside a replication of your spreadsheet.
Your company is looking to build a new incinerator that will generate electricity by burning household waste and thereby diverting trash from landfills. Someone in your office recommended calling this incinerator “The Big Kahuna” and no one else had any better ideas.
Each year you operate, you will generate electricity worth $1.5 million, and incur expenses of $400,000. Your incinerator will go online halfway through 2026, and will stop operations at the end of 2035 (i.e., 2026 will only have half the revenue and half the expenses but 2035 will have a full year).
The incinerator costs $3 million dollars, and you will finance its purchase with a bank loan offered at 6.5% annual interest compounded annually and 20% down, paid back over the next ten years (down payment in 2025, payments starting in 2026).
Incinerators like this one have a seven-year recovery period using the MACRS declining balance method. You also expect to salvage the equipment for 500,000 at the end of 2035. You pay a corporate tax rate of 28% on your taxable income, and your company has a MARR of 12%.
- Calculate the NPV of this project using a 12% discount rate.
- Calculate the IRR of this project.
Range of answers
If you have done this problem correctly, you should get an NPV in the neighborhood of $2.5 Million with an IRR between 50% and 100%.
HW 27: Self-financing the Big Kahuna
When you are about to finance the loan, one of the members of your board calls you up and says he would rather the company self-finance the incinerator purchase, to keep the company out of debt. Is this a good idea?
Tip
The best way to solve this problem is to simply copy your spreadsheet from the previous problem, and change it so that the entire cost of the incinerator comes up front, and remove the finance payments. Calculate the NPV and IRR, and use that information to tell your board member what you think the company should do.
Problem Set: Project Assessment
HW 28: Arizona Traffic Projects
You are evaluating the profitability of different traffic projects for the Arizona Department of Transportation (AzDOT). You assume that these projects will last a long time, and for simplicity you assume that the benefits can be projected for perpetuity (forever). The construction costs, and the annual returns from each project are listed in the table below. Assume that construction occurs at age 0 of the project, and the returns occur starting in year 1 for perpetuity.
| Project | Construction Cost | Annual Returns [M$] | Present Value (Returns) | Net Benefits | B/C | IRR |
|---|---|---|---|---|---|---|
| A | 20 | 1.8 | ||||
| B | 82 | 6.0 | ||||
| C | 120 | 10.0 | ||||
| D | 140 | 10.5 |
AzDOT uses a discount rate of 4% for all projects.
- Complete the table with these calculations. Show your work with an example calculation.
- Calculate the present value of the returns
- Calculate the net benefits
- Calculate the benefit/cost ratio
- Calculate the IRR (%)
Assume that projects A through D are for different independent traffic projects throughout Arizona. Your supervisor asks you to recommend which projects should be built this year given a budget of $140 Million. Which project(s) do you recommend? Which financial metric did you use to develop your recommendation? Hint: See the “Think” box in Section 4.7.1.
Assume that projects A through D are different alternatives for the same traffic project. This means that you can only do one of the projects. Again you have a budget for this project of $140 Million. Your supervisor asks you to recommend one project. Which financial metric do you recommend to use? Why? What are your net benefits?
HW 29: Traffic Engineer in Provo City
You are a traffic engineer for Provo City. You recently conducted a safety analysis of pedestrian crossings. One of the pedestrian crossings was identified as University Avenue between the old Provo High school and the BYU Indoor training facility.
The pedestrian fatality rate was estimated to be 0.01 fatalities per 100,000 pedestrians. You estimate that 200,000 pedestrians will use the pedestrian crossing per year. Assume that Provo City uses a value of a statistical life of $11,000,000.
You have developed two different proposals for the city. Option 1 is an elevated walkway, and Option 2 is an improved crosswalk with flashing lights. You have conducted a safety analysis of both options. Option 1 is the safest option, but the most expensive. Option 2 improves the safety, but is much less expensive. The lifetime of both projects is assumed to be 30 years.
| Project | Fatality Probability per 100,000 Pedestrians | Construction Costs |
|---|---|---|
| baseline | 0.0100 | 0 |
| Option 1 | 0.0010 | 4,000,000 |
| Option 2 | 0.0075 | 600,000 |
- Calculate the annual cost of the risk of pedestrian fatalities in dollars, assuming 200,000 annual pedestrians, estimated pedestrian fatality rates, and the value of a statistical life. From the baseline scenario, and the two proposals. Use the definition of risk found in Equation 4.35
- Calculate the benefits for each of the two proposals as the reduced risk of pedestrian fatalities over the 30-year life-time of the pedestrian crossings. Calculate the benefits as the reduction in risk from the baseline scenario. Calculate the present value of benefits over the 30-year lifetime of the pedestrian crossings. Use a discount rate of 2%.9
- Calculate the net benefits (Benefits – Construction Costs) for the lifetime of the project. Which project has the higher net benefits?
- Calculate the benefit/cost ratio for the lifetime of the project. Which project has a higher benefit/cost ratio?
- From the financial perspective, are both options worth considering? Why?
- Which project would you recommend? Why?
Problem Set: Multi-criteria
For example, tractor units have a recovery period of 3 years. Vehicles have a recovery periods of 5 years. Office furniture, railroad tracks, and natural gas lines are 7 years, land improvements, electric transmission properties are 15 years. Water treatment and water distribution properties are 25-years. As you can see the recovery periods only seem somewhat related to the actual life-time of the assets.(IRS 2024b)↩︎
There are also formulas for gradients that grow by less than a uniform amount. We will not cover these in this class↩︎
You can also see this as \(F/P = (1 + i)^n\), where the factor you want is isolated by the division.↩︎
There are many more tools than can be listed here, and these descriptions are perhaps dangerously oversimplified. But this gives a decent overview for undergraduate engineers.↩︎
A complete discussion of macroeconomic policy in setting and governing interest rates including the role of central banks is outside the scope of this class and the expertise of your instructor.↩︎
This isn’t how PhDs work in engineering, where your tuition is usually paid by your research grants.↩︎
This is sometimes called a Kepner-Tregoe (KT) matrix after the authors who proposed it as a rational process for decision making (Kepner and Tregoe 1981).↩︎
When we conduct a lifecycle analysis, shouldn’t we calculate the future value of cost and benefits to account for inflation, and then discount it back to the present value? In other words, if a VSL is worth $11 million today, shouldn’t it be worth even more in the future? The White House’s guidance on conducting lifecycle analysis recommends using constant-dollar values for future costs, and use a discount rate that has the inflation effect removed, called the real discount rate. The nominal discount rate is an estimate of the time-value of money, including the impact of inflation. For regulatory analysis, the White House recommends estimating the nominal discount rate from US treasury returns (OMB 2023). The real discount rate is calculated by subtracting the impact of inflation rate from the nominal discount rate. For example, if the nominal discount rate is 4%, and inflation is 2%, then the real discount rate is approximately 2% (See Equation 4.30). The net present value will be the same if your future costs include an inflation rate of 2%, and you discount using the nominal rate of 4%, as a case where future costs are expressed in constant-dollars, and you discount back to the future using the 2% real discount rate.↩︎